Análisis metodológico del esfuerzo normal σyy basado en deflexión elástica

Alejandro Molina; Manuel Román Piña-Monarrez; Servio Tulio de la Cruz-Cháidez

doi:10.37636/recit.v24166180

Authors

Alejandro Molina Department of Industrial Engineering and Manufacturing, of the Institute of Engineering and Technology (IIT) of the Autonomous University of Ciudad Juárez (UACJ), Cd. Juárez, Chihuahua, Mexico. https://orcid.org/0000-0002-1945-7727
Manuel Román Piña-Monarrez Department of Industrial Engineering and Manufacturing, of the Institute of Engineering and Technology (IIT) of the Autonomous University of Ciudad Juárez (UACJ), Cd. Juárez, Chihuahua, Mexico. https://orcid.org/0000-0002-2243-3400
Servio Tulio de la Cruz-Cháidez Department of Civil Engineering, Institute of Engineering and Technology (IIT) of the Autonomous University of Ciudad Juárez (UACJ), Cd. Juárez, Chihuahua, Mexico. https://orcid.org/0000-0003-0392-2097

DOI:

https://doi.org/10.37636/recit.v24166180

Keywords:

Static analysis, Normal efforts, Main efforts, Resistance analysis, Fatigue

Abstract

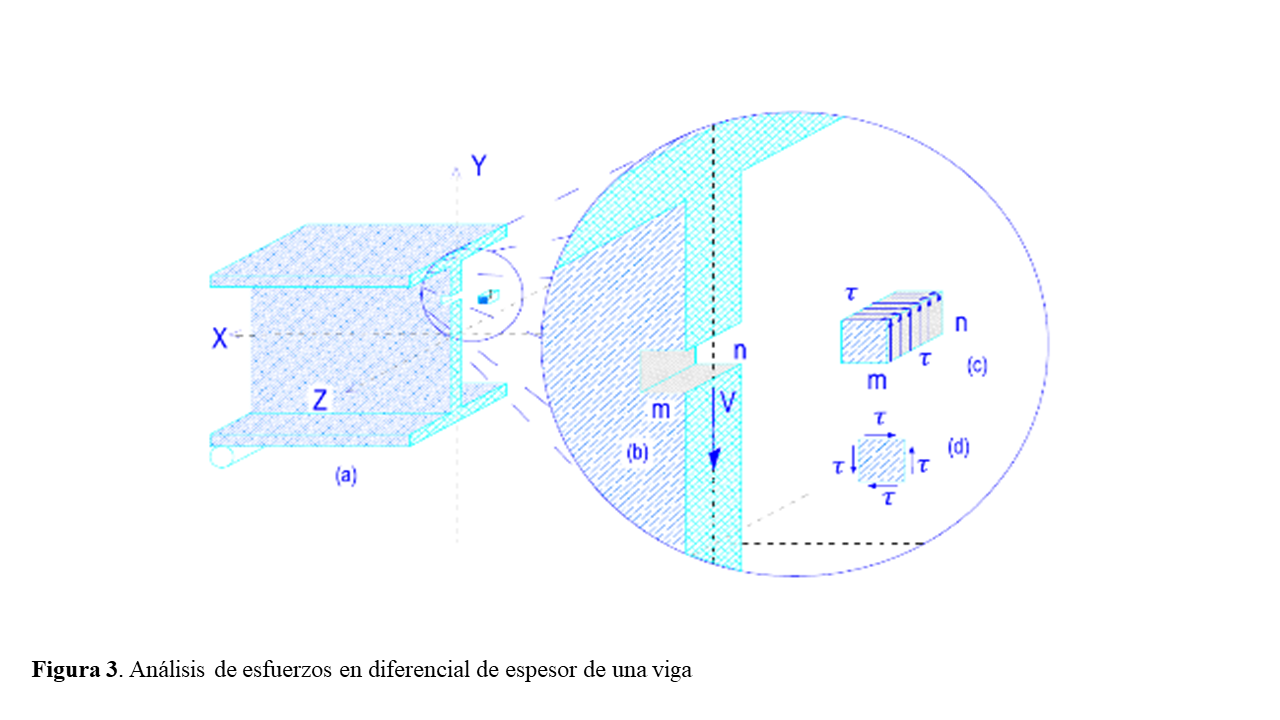
The problem in determining the normal stresses (σ_x, σ_y, τ_xy) in a cross section using elastic deflection as a base, is based on the fact that the existing methodologies still have deficiencies in their analysis. The article presents an analysis of the normal stresses (σ_x, σ_y, τ_xy) developed from the applied loads on the structural element and the development of an application case. Also, since the deflection of an element depends on the applied loads, then the stress analysis is based on the elastic deflection of the structural component. In addition, the selection of the structural element is based on the design regulations for beams for structural components. On the other hand, the analysis of the material to make a design of a structural component is also presented in this article. Likewise, the material will show wear due to the applied loads, then a fatigue analysis based on normal stresses is performed.

Downloads

Download data is not yet available.

References

R. G. Budynas and J. K. Nisbett, Shigley's mechanical engineering design, vol. 8. McGraw-Hill New York, 2008. https://books.google.com.mx/books/about/Shigley_s_Mechanical_Engineering_Design.html?id=eT1DPgAACAAJ&redir_esc=y.

D. Kececioglu, Robust engineering design-by-reliability with emphasis on mechanical components & structural reliability, vol. 1. DEStech Publications, Inc, 2003. https://doi.org/10.4271/2003-01-0141. DOI: https://doi.org/10.4271/2003-01-0141

Y.-L. Lee, J. Pan, R. Hathaway, and M. Barkey, Fatigue testing and analysis: theory and practice, vol. 13. Butterworth-Heinemann, 2005. https://www.sciencedirect.com/book/9780750677196/fatigue-testing-and-analysis.

E. Castillo and A. Fernández-Canteli, "A general regression model for lifetime evaluation and prediction," Int. J. Fract., vol. 107, no. 2, pp. 117-137, 2001. https://doi.org/10.1023/A:1007624803955. DOI: https://doi.org/10.1023/A:1007624803955

M. R. Piña-Monarrez, "Weibull stress distribution for static mechanical stress and its stress/strength analysis," Qual. Reliab. Eng. Int., vol. 34, no. 2, pp. 229-244, Dec. 2017. https://doi.org/10.1002/qre.2251. DOI: https://doi.org/10.1002/qre.2251

S. Timoshenko, "Resistencia de Materiales, Segunda Parte." ESPASA-CALPE SA, 1957. http://cj000528.ferozo.com/cordoba/taller1/DEtaller1timoshendo.pdf.

J. M. Gere and S. Timoshenko, "Mechanics of Materials; Brooks," Cole, Pacific Grove, CA, pp. 815-839, 2001. http://iusnews.ir/images/upfiles/20150313/moghavemat%20masaleh.pdf.

M. Cervera Ruiz and E. I. Blanco Díaz, "Mecánica de estructuras." Edicions UPC, 2002. http://cervera.rmee.upc.edu/libros/Mec%C3%A1nica%20de%20Estructuras.pdf.

A. C. Ugural and S. K. Fenster, Advanced mechanics of materials and applied elasticity. Pearson Education, 2011. https://books.google.com/books/about/Advanced_Mechanics_of_Materials_and_Appl.html?id=vLqkydSJ8vEC.

V. D. Da Silva, Mechanics and strength of materials. Springer Science & Business Media, 2005. https://doi.org/10.1007/3-540-30813-X. DOI: https://doi.org/10.1007/3-540-30813-X

X.-T. He, P. Xu, J.-Y. Sun, and Z.-L. Zheng, "Analytical solutions for bending curved beams with different moduli in tension and compression," Mech. Adv. Mater. Struct., vol. 22, no. 5, pp. 325-337, 2015. https://doi.org/10.1080/15376494.2012.736053. DOI: https://doi.org/10.1080/15376494.2012.736053

J. Liang, Z. Ding, and J. Li, "A probabilistic analyzed method for concrete fatigue life," Probabilistic Eng. Mech., vol. 49, pp. 13-21, 2017. https://doi.org/10.1016/j.probengmech.2017.08.002. DOI: https://doi.org/10.1016/j.probengmech.2017.08.002

Q. Guo, X. Guo, J. Fan, R. Syed, and C. Wu, "An energy method for rapid evaluation of high-cycle fatigue parameters based on intrinsic dissipation," Int. J. Fatigue, vol. 80, pp. 136-144, 2015. https://doi.org/10.1016/j.ijfatigue.2015.04.016. DOI: https://doi.org/10.1016/j.ijfatigue.2015.04.016

S. Ma and H. Yuan, "A continuum damage model for multi-axial low cycle fatigue of porous sintered metals based on the critical plane concept," Mech. Mater., vol. 104, pp. 13-25, 2017. https://doi.org/10.1016/j.mechmat.2016.09.013. DOI: https://doi.org/10.1016/j.mechmat.2016.09.013

Y. J. Kim and K. A. Harries, "Fatigue behavior of damaged steel beams repaired with CFRP strips," Eng. Struct., vol. 33, no. 5, pp. 1491-1502, 2011. https://doi.org/10.1016/j.engstruct.2011.01.019. DOI: https://doi.org/10.1016/j.engstruct.2011.01.019

J. Huang, M.-L. Pastor, C. Garnier, and X. Gong, "Rapid evaluation of fatigue limit on thermographic data analysis," Int. J. Fatigue, vol. 104, pp. 293-301, 2017. https://doi.org/10.1016/j.ijfatigue.2017.07.029. DOI: https://doi.org/10.1016/j.ijfatigue.2017.07.029

A. Molina, M. R. Piña-Monarrez, and J. M. Barraza-Contreras, "Stress-Strength Reliability Based Design Analysis to W-beam using a probabilistic approach.," En proceso publicación, 2019.

J. Xu, M. Huo, and R. Xia, "Effect of cyclic plastic strain and flow stress on low cycle fatigue life of 316L (N) stainless steel," Mech. Mater., vol. 114, pp. 134-141, 2017. https://doi.org/10.1016/j.mechmat.2017.07.014. DOI: https://doi.org/10.1016/j.mechmat.2017.07.014

X. Gao, R. H. Dodds Jr, R. L. Tregoning, and J. A. Joyce, "Weibull stress model for cleavage fracture under high‐rate loading," Fatigue Fract. Eng. Mater. Struct., vol. 24, no. 8, pp. 551-564, 2001. https://doi.org/10.1046/j.1460-2695.2001.00421.x. DOI: https://doi.org/10.1046/j.1460-2695.2001.00421.x

A. S. Design, "Specification for structural steel buildings," AISC, December, vol. 27, 1999. https://www.aisc.org/Specification-for-Structural-Steel-Buildings-ANSIAISC-360-16-1.

J. McCormac, Diseño de estructuras de acero. Alfaomega Grupo Editor, 2016. https://www.alfaomega.com.mx/default/dise-o-de-estructuras-de-acero-5a-ed-5499.html.

Http://www.matweb.com, "ASTM A570 Steel, grade 50." [Online]. Available: http://www.matweb.com/search/datasheet.aspx?MatGUID=e499c7dc3e9545d1b8a3766dcffd6139&ckck=1.

A. C. Ugural, Mechanical Design: An Integrated Approach. McGraw-Hill/Higher Education, 2004.

https://www.pearson.com/store/p/machine-design-an-integrated-approach/P100002946749.