Exact volume calculation inside tilted cylindrical container as a function of the fill level
DOI:
https://doi.org/10.37636/recit.v7n2e349Keywords:
Cylindrical recipe, Fill level, Exact Volume, Tilt container, First wet height, Second wet heightAbstract
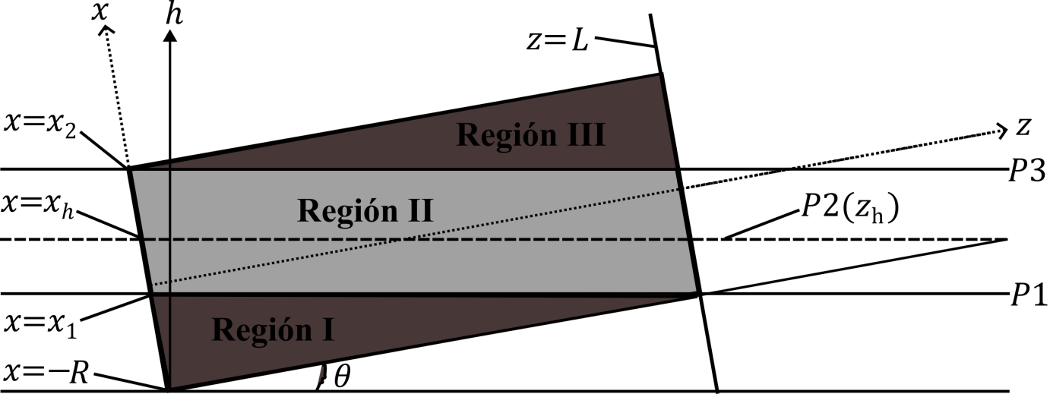
In the present work math expressions to determine the exact volume inside a tilted cylindrical recipe (container) as a function of the fill level were obtained. The tilt container is given by the angle between the horizontal and the axis symmetry of the cylinder. Formulations are developed for three cases: i) the limits of the filled level are between the lowest point in the container and the corresponding level where a horizontal plane reaches the first wet height, ii) the filled level is given by the liquid height limited by the horizontal planes corresponding to the first and second wet heights, respectively; iii) the filled level starts at the horizontal plane corresponding to the second wet height and ending with the fill full of the container. The math expressions give the exact volume inside the container as a function of the known parameters of radius, length, and tilt of the cylinder, and the variable parameter represented by the filled level. Finally, these expressions are applied using critical and regular dimensions of the recipe in the substance’s transportation, the graph volume vs filled level shows a behavior like a sinusoidal function. The results obtained lead to best practices in the design of cylindrical containers for the transport of substances and the implementation of security protocols.
Downloads
References
J. Sun, «The discussion of horizontal tank volume about the problematic point of the verification and calculation», Pet. Prod. Appl. Res., vol. 18, n.o 5, pp. 20-24, 2000.
NORMA Oficial Mexicana, «Listado de las substancias y materiales peligrosos más usualmente transportados». 2011.
American Petroleum Institute, «Standard Method for Measurement and Calibration of Hori-zontal Tanks». Washington D. C.: American Petroleum Institute, 1965.
J. Guan y H. Zhao, «Practical methods of oil volume calibration of horizontal storage tank», Metrol. Meas. Tech., vol. 31, n.o 3, pp. 21-36, 2004.
American Petroleum Institute, «Chapter 2.2E: Petroleum and Liquid Petroleum Products - Calibration of Horizontal Cylindrical Tanks - Part 1: Manual Methods, First Edition, Includes Errata (2009)», en Manual of Petroleum Measurement Standards, Washington D. C.: American Petroleum Institute, 2014.
C. Li, W. Zhow, J. Lu, y T. Liang, «The function relation of remain liquid volume and oil height of storage tank», Petro-Chem. Equip., vol. 6, pp. 25-27, 2001.
D. G. Zill, Matemáticas 3: Cálculo de varias variables, 2a ed. McGraw Hill/Interamericana, 2015.
Cálculo de varias variables. Ciudad de México: Cengage Learning, 2023.
L. Leithold, El cálculo, Séptima edición, Trigésima tercera reimpresión. México D.F.: Oxford University Press, 1998.
J. Stewart, Cálculo de varias variables: trascendentes tempranas, Octava edición. México: Cengage Learning, 2018.
Y. Nievergelt, «Calculus Measures Tank Capacity and Avoids Oil Spills», Coll. Math. J., vol. 25, n.o 2, pp. 132-136, mar. 1994, doi: 10.1080/07468342.1994.11973596. DOI: https://doi.org/10.1080/07468342.1994.11973596
L. Alonso-Preciado, W. Suárez-Piña, y J. Zaldívar-Chacón, «Corrección de los errores por inclinación en la calibración de tanques cilíndricos nominalmente horizontales», Bol. Científico Téc. INIMET, vol. 2, pp. 7-14, 2008.
NORMA CUBANA 846: 2011, «Métodos y medios de verificación», en Tanques cilíndricos horizontales, 1981.
Z. Li, «The calculation of horizontal storage tank volume with elliptic cylinder type», Math. Pract. Theory, vol. 2, pp. 17-26, 1997.
W. Khaisongkram y D. Banjerdpongchai, «A combined geometric-volumetric calibration of inclined cylindrical underground storage tanks using the regularized least-squares method.», International Conference on Control Applications, 2004, pp. 1515-1520, 2004.
X. G. Pan, «Measurement and Calculation of Tilt Horizontal Tank Volume», J. Oil Gas Storage Transp., vol. 6, n.o 6, pp. 47-50, 1987.
T. J. Tian, «Volume Calculation of the Straight Cylindric Part of Tihed Horizontal Tank», J. Mod. Meas. Test, vol. 5, pp. 32-36, 1999.
W. Xie, X. Wang, H. Cui, y J. Chen, «Optimization Model of Oil-Volume Marking with Tilted Oil Tank», Open J. Optim., vol. 01, n.o 02, pp. 20-24, 2012, doi: 10.4236/ojop.2012.12004. DOI: https://doi.org/10.4236/ojop.2012.12004
E. Q. Gao y P. Y. Feng, «Calculation of the Reserve of Horizontal Cylindrical Oil Storage Tank Declined Fiting at Different Liquid Level», J. Shandong Metall., vol. 1, pp. 26-28, 1998.
J. Dou, Y. Mai, Z. Chen, y L. Wang, «Model of the identification of oil tank’s position and the calibration of tank capacity table», Pure Appl. Math., vol. 27, n.o 6, pp. 829-840.
S. Ou, J. Wang, y S. Han, «Model of the identification of oil tank’s position and the calibration of tank capacity table», China Pet. Chem. Stand. Qual., vol. 31, n.o 4, pp. 25-26.
C. Li, Y. Yuan, L. Song, Y. Tan, y G. Wang, «Mathematical Model Based on BP Neural Network Algorithm for the Deflection Identification of Storage Tank and Calibration of Tank Capacity Chart», Abstr. Appl. Anal., vol. 2013, pp. 1-13, 2013, doi: 10.1155/2013/923036. DOI: https://doi.org/10.1155/2013/923036
International Standardization Organization, «Petroleum and liquid petroleum products—Calibration of horizontal cylindrical tanks Part 1: Manual methods.», 2002.
A. Preciado, L. Yamí, W. Suárez-Piña, y J. A. Saldívar-Chacón, «CORRECCIÓN DE LOS ERRORES POR INCLINACIÓN EN LA CALIBRACIÓN DE TANQUES CILÍNDRICOS NOMINALMENTE HORIZONTALES», vol. 2, pp. 7-14, 2008.
International Standardization Organization, «Part 1: Manual methods», en ISO 12917 Petroleum and liquid petroleum products -- Calibration of horizontal cylindrical tanks, Ginebra: ISO, 2002.
R. A. Mora Casal y J. R. Mora Casal, «Implementación de tablas de corrección al volumen del tanque cilíndrico horizontal inclinado, compatibles con la norma ISO 12917:2017», Rev. Ing., vol. 29, n.o 2, jul. 2019, doi: 10.15517/ri.v29i2.36211. DOI: https://doi.org/10.15517/ri.v29i2.36211
ENGINEER STANDARDS, «API 2551», en Standard Method for Measurement and Calibration of Horizontal Tanks, GEORGIA SOUTHERN UNIVERSITY.
W. L. Coats, «Calibration of Cylindrical Tanks with Axis Inclined», J. Inst. Petro-Leum, vol. 34, n.o 297, pp. 627-646, 1948.
NORMA Oficial Mexicana, «SOBRE EL PESO Y DIMENSIONES MÁXIMAS CON LOS QUE PUEDEN CIRCULAR LOS VEHÍCULOS DE AUTOTRANSPORTE QUE TRANSITAN EN LAS VÍAS GENERALES DE COMUNICACIÓN DE JURISDICCIÓN FEDERAL». 2017.
S. L. Salas, G. J. Etgen, y E. Hille, Calculus una y varias variables, 4a. ed. Barcelona (España): Editorial Reverté, 2011.
Published
How to Cite
Issue
Section
Categories
License
Copyright (c) 2024 Michelle Guadalupe Salas-Flores, David Gasca-Figueroa, Francisco Javier García-Rodríguez, José Alfredo Ramos-Beltrán, Jorge Macias-Aboytes, Jafet Gassen Tula-Maldonado

This work is licensed under a Creative Commons Attribution 4.0 International License.
The authors who publish in this journal accept the following conditions:
The authors retain the copyright and assign to the journal the right of the first publication, with the work registered with the Creative Commons Attribution license 4.0, which allows third parties to use what is published as long as they mention the authorship of the work and the first publication in this magazine.
Authors may make other independent and additional contractual agreements for the non-exclusive distribution of the version of the article published in this journal (eg, include it in an institutional repository or publish it in a book) as long as they clearly indicate that the work it was first published in this magazine.
Authors are allowed and encouraged to share their work online (for example: in institutional repositories or personal web pages) before and during the manuscript submission process, as it can lead to productive exchanges, greater and more quick citation of published work (see The Effect of Open Access).











