Dimensionality and geometry effects on a quantum carnot engine efficiency

Authors

  • Hiram Kalid Herrera Alcantar Faculty of Sciences, Autonomous University of Baja California, Ensenada, Baja California, Mexico
  • José Carlos Carvajal García Faculty of Sciences, Autonomous University of Baja California, Ensenada, Baja California, Mexico
  • Osvaldo Rosales Pérez Faculty of Sciences, Autonomous University of Baja California, Ensenada, Baja California, Mexico
  • Rubén Cesar Villarreal-Sánchez Faculty of Engineering, Architecture and Design, Autonomous University of Baja California, Ensenada-Tijuana 3917 transpeninsular highway, Playitas neighborhood, Ensenada, Baja California, Mexico https://orcid.org/0000-0002-5395-580X
  • Priscilla Elizabeth Iglesias-Vázquez Faculty of Sciences, Autonomous University of Baja California, Ensenada, Baja California, Mexico

DOI:

https://doi.org/10.37636/recit.v214548

Keywords:

Carnot cycle, Heat engine, Quantum confinement.

Abstract

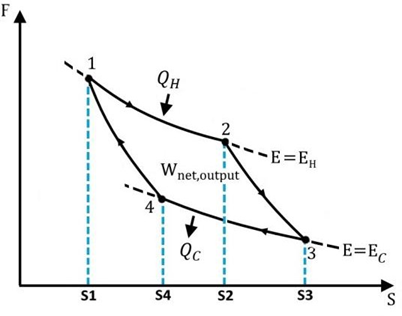
We calculate the efficiency of a quantum Carnot cycle for a particle confined in two different infinite potential wells, a cylindrical potential well of variable radius and a two-dimensional square potential well with a periodicity in one of it sides. We find that the efficiency depends directly on the dimensionality and geometry of the well that confined the particle.

Downloads

Download data is not yet available.

References

S. Carnot, “Réflexions sur la puissance motrice du feu”. Paris, Annales scientifiques de l’É.N.S. 2e série, tome 1, p. 393-457, 1872. http://www.numdam.org/item?id=ASENS_1872_2_1__393_0 DOI: https://doi.org/10.24033/asens.88

J. E. Geusic, E. O. Schulz-DuBios, and H. E. D. Scovil, “Quantum equivalent of the Carnot cycle,” Phys. Rev., vol. 156(2), pp. 343. 1967. https://doi.org/10.1103/PhysRev.156.343 DOI: https://doi.org/10.1103/PhysRev.156.343

C. M. Bender, D. C. Brody, and B. K. Meister, “Quantum mechanical Carnot engine,” J. Phys. A. Math. Gen., vol. 33, no. 24, pp. 4427–4436, 2000. https://doi.org/10.1088/0305-4470/33/24/302 DOI: https://doi.org/10.1088/0305-4470/33/24/302

H. T. Quan, Y. Liu, C. P. Sun, and F. Nori, “Quantum thermodynamic cycles and quantum heat engines,” Phys. Rev. E, vol. 76, no. 3, p. 31105, Sep. 2007. https://doi.org/10.1103/PhysRevE.76.031105 DOI: https://doi.org/10.1103/PhysRevE.76.031105

H. T. Quan, “Quantum thermodynamic cycles and quantum heat engines. II.,” Phys. Rev. E, vol. 79, no. 4, p. 41129, 2009. https://doi.org/10.1103/PhysRevE.79.041129 DOI: https://doi.org/10.1103/PhysRevE.79.041129

T. D. Kieu, “The second law, Maxwell's demon, and work derivable from quantum heat engines,” Phys. Rev. Lett., vol. 93(14), pp.140403. 2004. https://doi.org/10.1103/PhysRevLett.93.140403 DOI: https://doi.org/10.1103/PhysRevLett.93.140403

S. Abe and S. Okuyama, “Similarity between quantum mechanics and thermodynamics: Entropy, temperature, and Carnot cycle,” Phys. Rev. E, vol. 83, no. 2, p. 21121, Feb. 2011. https://doi.org/10.1103/PhysRevE.83.021121 DOI: https://doi.org/10.1103/PhysRevE.83.021121

J. Wang, J. He, and Z. Wu, “Efficiency at maximum power output of quantum heat engines under finite-time operation,” Phys. Rev. E, vol. 85, no. 3, p. 31145, Mar. 2012. https://doi.org/10.1103/PhysRevE.85.031145 DOI: https://doi.org/10.1103/PhysRevE.85.031145

S. Abe, “General formula for the efficiency of quantum-mechanical analog of the Carnot engine,” Entropy, vol. 15(4), pp. 1408. 2013. https://doi.org/10.3390/e15041408 DOI: https://doi.org/10.3390/e15041408

B. Zwiebach, A First Course in String Theory, 2nd ed. Cambridge: Cambridge University Press, 2009. https://doi.org/10.1017/CBO9780511841620 DOI: https://doi.org/10.1017/CBO9780511841620

P. P. Hofer, J.-R. Souquet, and A. A. Clerk, “Quantum heat engine based on photon-assisted Cooper pair tunneling,” Phys. Rev. B, vol. 93, no. 4, p. 41418, 2016. https://doi.org/10.1103/PhysRevB.93.041418 DOI: https://doi.org/10.1103/PhysRevB.93.041418

P. P. Hofer, J. B. Brask, M. Perarnau-Llobet, and N. Brunner, “Quantum Thermal Machine as a Thermometer,” Phys. Rev. Lett., vol. 119, no. 9, p. 90603, Sep. 2017. https://doi.org/10.1103/PhysRevLett.119.090603 DOI: https://doi.org/10.1103/PhysRevLett.119.090603

M. Campisi and R. Fazio, “The power of a critical heat engine,” Nat. Commun., vol. 7, no. 1, p. 11895, 2016. https://doi.org/10.1038/ncomms11895 DOI: https://doi.org/10.1038/ncomms11895

R. Shankar, “Principles of quantum mechanics”. New York: Plenum Press. 1994. https://doi.org/10.1007/978-1-4757-0576-8 DOI: https://doi.org/10.1007/978-1-4757-0576-8

Illustration of a four-step quantum Carnot cycle, where S denote the length of the different types of potential wells and F is the force exerted on the wall of the wells

Downloads

Published

2019-02-27

How to Cite

Herrera Alcantar, H. K., Carvajal García, J. C., Rosales Pérez, O., Villarreal-Sánchez, R. C., & Iglesias-Vázquez, P. E. (2019). Dimensionality and geometry effects on a quantum carnot engine efficiency. Revista De Ciencias Tecnológicas, 2(1), 45–48. https://doi.org/10.37636/recit.v214548

Issue

Vol. 2 No. 1 (2019): January-March

Section

Case studies

Categories

License

Copyright (c) 2019 Herrera Alcantar Hiram Kalid, Carvajal García José Carlos, Rosales Pérez Osvaldo, Villarreal Sánchez Rubén Cesar, Iglesias-Vázquez Priscilla Elizabeth​

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The authors who publish in this journal accept the following conditions:


The authors retain the copyright and assign to the journal the right of the first publication, with the work registered with the Creative Commons Attribution license 4.0, which allows third parties to use what is published as long as they mention the authorship of the work and the first publication in this magazine.


Authors may make other independent and additional contractual agreements for the non-exclusive distribution of the version of the article published in this journal (eg, include it in an institutional repository or publish it in a book) as long as they clearly indicate that the work it was first published in this magazine.


Authors are allowed and encouraged to share their work online (for example: in institutional repositories or personal web pages) before and during the manuscript submission process, as it can lead to productive exchanges, greater and more quick citation of published work (see The Effect of Open Access).

Crossref
Scopus
Google Scholar
Europe PMC